The height of a triangle is three more than two times the base, can be translated algebraically as
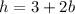
If the area of the triangle is 45 square feet, then we can have the equation
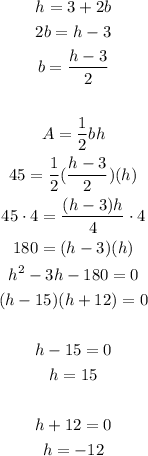
Disregarding the negative values for h, we use h = 15. And then use this to solve for the base
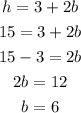
The dimensions therefore of the triangle is height of 15 feet, and a base of 6 feet.