Question

Jamarie is serving both vegan chocolate cake and vegan vanilla ice cream cake at her birthday party this weekend. The chocolate cake has a diameter of 14 inches and will be cut into slices that measure 20 degrees each. The vanilla ice cream cake has a diameter of 10 inches and is cut into 8 equal slices. You want to eat lots of cake but want to be polite and only take one slice. Which cake should you choose, and why?Vegan Vanilla Ice Cream Cake because its slices are bigger.Vegan Chocolate Cake because its slices are bigger.Neither, because I'm neither vegan nor do I want to be a diabetic,

Answer 1

Vegan Vanilla Ice Cream Cake because its slices are bigger.

Step-by-step explanation:

In order to compare, we find the area of each slice.

The slice is in the shape of the sector of a circle.

`\begin{gathered} \text{Area of a sector =}(\theta)/(360)*\pi r^2 \\ \theta=\text{Central Angle} \end{gathered}`

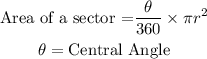
Chocolate cake

Diameter = 14 inches

`\begin{gathered} Radius=Diameter/2 \\ =14/2 \\ =7\text{ inches} \end{gathered}`

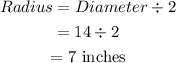
Each slice will measure 20 degrees, therefore:

The central angle = 20 degrees.

`\begin{gathered} \text{Area of a sector =}(20)/(360)*\pi*7^2 \\ =(49)/(18)\pi \\ =2.72\pi\text{ square inches} \end{gathered}`

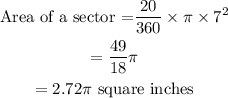
Vanilla ice cream cake

Diameter = 10 inches

`\begin{gathered} Radius=10/2 \\ =5\text{ inches} \end{gathered}`

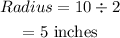
Since the vanilla cake will be cut into 8 equal parts, the central angle will be:

`\theta=(360\degree)/(8)=45\degree`

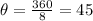
The area of one slice of the vanilla cake will be:

`\begin{gathered} \text{Area =}(45)/(360)*\pi*5^2 \\ =(25)/(8)\pi \\ =3.125\pi\text{ square inches} \end{gathered}`

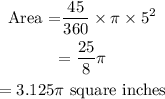
Comparison

The area of one slice of vanilla cake is bigger,

Therefore, the individual should choose Vegan Vanilla Ice Cream Cake because its slices are bigger.