Question

Mr. Haley is leaning a ladder against the side of his house to repair the roof.

The top of the ladder reaches the roof, which is 12 feet high.
The base of the ladder is 9 feet away from the house, where Mr. Haley's son is holding it steady,
What is the length of the ladder?

Answer 1

15 ft

Step-by-step explanation:

We can represent the situation with the following figure

Therefore, the length of the ladder is the hypotenuse of the right triangle formed, where the legs are 12 ft and 9 ft. Using the Pythagorean theorem, we can calculate the length of the ladder as

`\begin{gathered} Ladder=√(12^2+9^2) \\ Ladder=√(144+81) \\ Ladder=√(225) \\ Ladder=15 \end{gathered}`

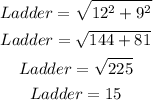
Therefore, the length of the ladder is 15 ft