Answer:
15 feet
Step-by-step explanation:
Let's go ahead and draw a sketch as seen below;
We can go ahead and solve for x using the Pythagorean theorem as seen below;

Recall that a quadratic equation in standard form is given as;

Comparing both equations, we can see that a = 2, b = -10, and c = -600
We'll go ahead and use the quadratic formula to solve for x as seen below;

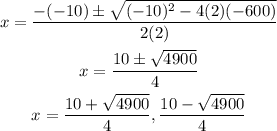

Since we're solving for distance, we'll go with the positive value of x which is 20. So x = 20 ft.
So the distance between the base of the ladder and the wall will be 15 ft (x - 5 = 20 - 5 = 15)