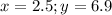Question

1. Calculate the components of a vector A in the x-y plane if its direction is 250 counterclockwise from the positive x-axis and its magnitude is 7.3 units.

Answer 1

`x=2.5;y=6.9`

Step-by-step explanation

We want to find the x and y components of vector A.

The direction of a vector in the x-y plane is:

`\theta=\tan ^(-1)((y)/(x))`

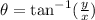
where x = x component; y = y component

Therefore, we have that:

`\begin{gathered} 250\degree=\tan ^(-1)((y)/(x)) \\ \Rightarrow\tan 250=(y)/(x) \\ \Rightarrow y=x\tan 250 \end{gathered}`

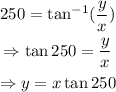
The magnitude of a vector is:

`|A|=\sqrt[]{x^2+y^2}`

Substitute the value of A and y obtained above into the equation:

`7.3=\sqrt[]{x^2+(x\tan250)^2}=\sqrt[]{x^2+x^2\tan ^2(250)}`

Solve for x in the equation:

`\begin{gathered} 7.3^2=x^2(1+\tan ^2(250)) \\ \Rightarrow x^2=(7.3^2)/(1+\tan ^2250) \\ x^2=(53.29)/(1+7.549)=(53.29)/(8.549) \\ x^2=6.2337 \\ \Rightarrow x=\sqrt[]{6.2337} \\ x\approx2.5 \end{gathered}`

Recall that:

`y=x\tan 250`

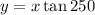
Substitute the value of x:

`\begin{gathered} y=2.5\tan 250 \\ y=6.9 \end{gathered}`

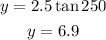
Therefore, the components are:

`x=2.5;y=6.9`