Answer
The volume of the container that the gas is in = 874.30 L
Step-by-step explanation
Given:
Moles, n = 3.2 mol
Pressure, P = 0.095 atm
Temperature, T = 43 °C = (43 + 273.15 K) = 316.15 K
Molar gas constant, R = 0.0821 atm•L/mol•K
What to find:
The volume of the container that the gas occupied.
Step-by-step solution:
The volume of the container that the gas occupied can be calculated using the ideal gas equation.
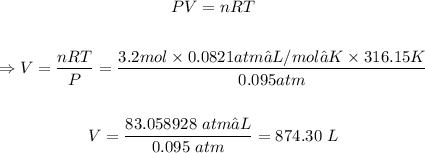
Therefore, the volume of the container that the gas occupied is 874.30 L