We have a circle represented by the equation:
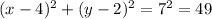
Any point that satisfy the equation of the circle lies in the circumference of the circle.
We can test each point by replacing the values of x and y in the equation by the coordinates of the point.
A) (-1,4)
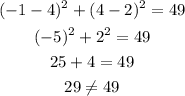
The point (-1,4) does not lie in the circle.
B) (8,3)
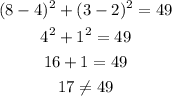
The point (8,3) does not lie in the circle.
C) (9,0)
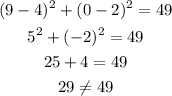
The point (9,0) does not lie in the circle.
D) (-2,2)
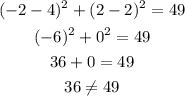
The point (-2,2) does not lie in the circle.