Question

Solve the equation. Then check your solution.4- 3/5(3a+4)=7A. -7.4B. -6C. 3D. -3

Answer 1

Answer: a = -3

Explanations:

The given equation is:

`4\text{ - }(3)/(5)(3a+4)\text{ = 7}`

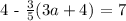
This can be re-written as:

`4-(3(3a+4))/(5)=7`

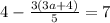
Collect like terms:

`\begin{gathered} -(3(3a+4))/(5)=\text{ 7 - 4} \\ -(3(3a+4))/(5)=\text{ 3} \end{gathered}`

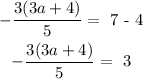
Cross multiply:

`\begin{gathered} -3(3a+4)\text{ = 3}*5 \\ -3(3a+4)=\text{ 15} \\ \text{Expand the bracket} \\ -9a\text{ - 12 = 15} \\ \text{Collect like terms} \\ -9a\text{ = 15 + 12} \\ -9a\text{ = 27} \\ \text{Divide both sides by -9} \\ (-9a)/(-9)=(27)/(-9) \\ a\text{ = -3} \end{gathered}`

To verify if the solution is correct, substitute a = -3 into the question given. If the Right Hand Side equals the Left Hand Side, then the solution is correct.

`\begin{gathered} 4-(3(3a+4))/(5)=\text{ 7} \\ \text{Substitute a = }-3 \\ 4\text{ - }(3(3(-3)+4))/(5)\text{ = 7} \\ 4\text{ - }(3(-9+4))/(5)\text{ = 7} \\ 4\text{ - }(3(-5))/(5)\text{ = 7} \\ 4\text{ - }(-15)/(5)=\text{ 7} \\ 4-(-3)=7 \\ 4+3=7 \\ 7=7 \end{gathered}`

Since the Left Hand Side = Right Hand Side = 7, the solution a = -3 is correct