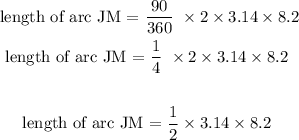Question

Find the length of arc JM. Use 3.14 for n.Round to the nearest tenth.16.4 miesЛKM[? ]miles

Answer 1

`\text{ length of arc JM = }12.9\text{ miles}`Step-by-step explanation:

arc MK = 90°

arc JMK = 180°

arc MK + arc JM = 180° (sum of arc in a semicircle)

90 + arc JM = 180

arc JM = 180 - 90

arc JM = 90°

To get the length of an arc, we will apply the formula:

`\text{length of an arc = }(\theta)/(360)*2\pi r`

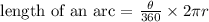
diameter = JK = 16.4 miles

diameter = 2(radius)

radius = diameter/2

radius = 16.4/2 = 8.2 miles

π = 3.14

Substitute the values:

`\begin{gathered} \text{length of arc JM = }(90)/(360)\text{ }*2*3.14*8.2 \\ \text{length of arc JM = }(1)/(4)\text{ }*2*3.14*8.2\text{ } \\ \\ \text{length of arc JM = }(1)/(2)*3.14*8.2\text{ } \end{gathered}`
`\begin{gathered} \text{length of arc JM = }12.874 \\ To\text{ the nearest tenth, length of arc JM = }12.9\text{ miles} \end{gathered}`