Explanation
We are given the following:

We are required to determine the following:
• The missing length of the lateral triangle.
,
• The lateral area.
,
• The single base area.
,
• The surface area of the figure.
We can obtain the missing length of the lateral triangle by using the Pythagorean theorem as follows:
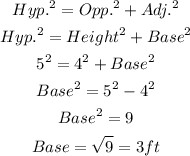
Hence, the missing length of the triangle (base) is 3ft.
Next, the lateral area can be determined as:

Hence, the lateral area is 61 square feet.
The base area can be calculated thus:
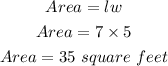
Hence, the single base area is 35 square feet.
Finally, we can calculate the surface area of the figure as:
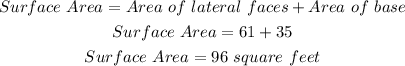
Hence, the surface area of the figure is 96 square feet.