Question

How do you solve the equations if the exponent is negative

Answer 1

A. 1/9

Step-by-step explanation

When we have an expression with a negative exponent we can solve it by "flipping" the fraction and solving with the positive exponent:

`\mleft((a)/(b)\mright)^(-c)=\mleft((b)/(a)\mright)^c`

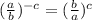
In this problem we have to find:

`f(-2)=3^(-2)`

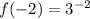
We can think of a whole number as a fraction whose denominator is 1:

`f(-2)=\mleft((3)/(1)\mright)^(-2)`

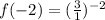
So we can use the trick:

`f(-2)=\mleft((1)/(3)\mright)^2`

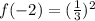
And solve:

`f(-2)=(1)/(3^2)=(1)/(9)`