We are given quadrilateral KLMN with vertices at K(0, 11), L(-9, 3), M(4, -8), and N(9, 4).
Also given is quadrilateral K'L'M'N' with vertices at K'(0, 5.5), L'(-4.5, 1.5), M'(2, -4), and N'(4.5, 2).
To find the scale factor used, we need to know by how much each side has grown or shrunk. We can get the length of KL and compare it K'L'.
We can use the distance formula for this:
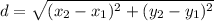
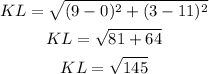

The scale factor used was:
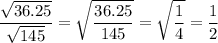
The answer is 1/2.