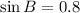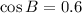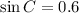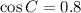The given triangle is
We get hypotenuse =BC=40 by observing the given triangle.
Consider the angle B.
For angle B, the Opposite side is AC=32 adjacent side AB =24.
We know that
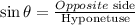
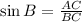
Substitute AC=32 and BC=40, we get

We know that
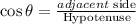
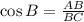
Substitute AB=24 and BC=40, we get
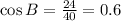
Consider the angle C.
For angle C, the Opposite side is AB=24 and the adjacent side AC=32.

Substitute AB=24 and BC=40, we get
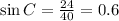

Substitute AC=32 and BC=40, we get
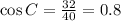
Hence the answers are