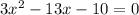Question

Write the equation of a quadratic in general form given two of its solutions.x= -2/3 ; x= 5

Answer 1

Given the roots/solutions of a quadratic equation as;

`x=(-2)/(3)\text{ and }x=5`

The general way of solving this kind of problem is by inserting/substituting it into the general form of factorized quadratic equation which is;

`\begin{gathered} (x-a)(x-b)=0 \\ \text{where a and b are the roots.} \end{gathered}`

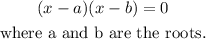
substituting the roots given in the question we have;

`\begin{gathered} (x-a)(x-b)=0 \\ a=(-2)/(3)\text{ and b}=5 \\ \text{Then;} \\ (x-(-2)/(3))(x-5)=0 \\ (x+(2)/(3))(x-5)=0 \end{gathered}`

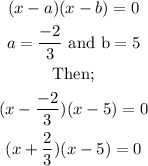
Then we can now expand to get the Quadratic equation.

`\begin{gathered} (x+(2)/(3))(x-5)=0 \\ x^2-5x+(2)/(3)x-(10)/(3)=0 \\ \text{multiplying through by 3, we have;} \\ 3x^2-15x+2x-10=0 \\ 3x^2-13x-10=0 \end{gathered}`

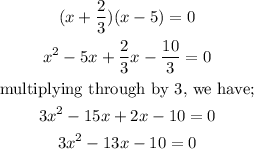
So, the quadratic equation with the roots/solutions x= -2/3 and x=5 is;

`3x^2-13x-10=0`