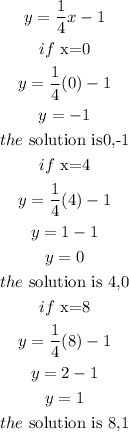Question

Determine 3 solutions to each of the following equations a. 3x-y=3b. Y=1/4x-1

Answer 1

In order to find solutions to the equations, we need to find ordered pairs that make the equality true, for that we give different values for x and solve the equation for y, like this:

A)

`\begin{gathered} 3x-y=3 \\ if\text{ }x=0 \\ 3(0)-y=3 \\ -y=3 \\ y=-3 \\ the\text{ solution is \lparen0,-3\rparen} \end{gathered}`

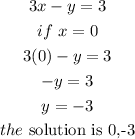
repeat with two different values for x

`\begin{gathered} if\text{ }x=3 \\ 3(3)-y=3 \\ 9-y=3 \\ -y=3-9 \\ -y=-6 \\ y=6 \\ the\text{ solution is \lparen3,6\rparen} \\ if\text{ }x=1 \\ 3(1)-y=3 \\ 3-y=3 \\ -y=3-3 \\ -y=0 \\ y=0 \\ the\text{ solution is \lparen1,0\rparen} \end{gathered}`

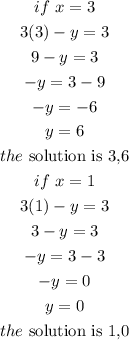
repeat the same procedure for part b

`\begin{gathered} y=(1)/(4)x-1 \\ if\text{ x=0} \\ y=(1)/(4)(0)-1 \\ y=-1 \\ the\text{ solution is\lparen0,-1\rparen} \\ if\text{ x=4} \\ y=(1)/(4)(4)-1 \\ y=1-1 \\ y=0 \\ the\text{ solution is \lparen4,0\rparen} \\ if\text{ x=8} \\ y=(1)/(4)(8)-1 \\ y=2-1 \\ y=1 \\ the\text{ solution is \lparen8,1\rparen} \end{gathered}`