Givens.
• The principal is 200,000.
,
• The interest rate is 14%.
,
• The time is 8 years.
,
• The compounding periods per year are n = 2.
Use the compound interest formula

Use the given values to find A.

But, A refers to the final amount including principal plus interest. Let's subtract it to find the amount of interest.
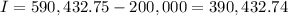
Therefore, the amount of compound interest earned is $390,432.74.