Question

Dilate the figure by the scale factor. Then enterthe new coordinates.A(0,2)K=10A'([?],[])1013.2 B'([][])C'([],[)B(,-2)C(-1,-4)

Answer 1

A dilation is a transformation that produces an image that is the same shape as the original, but is a different size.

The coordinates of the points A, B, and C given in the question are:

`\begin{gathered} A=0,2 \\ B=3,-2 \\ C=-1,-4 \end{gathered}`

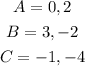
If an object with coordinates A (x,y) is dilated by a scale factor of k, the transformation will be

`A(x,y)\Rightarrow A^(\prime)(k* x,k* y)`

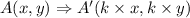
Applying this rule,

we have the values of

K=10

A=(0,2)

B=(3,-2)

C=(-1,-4)

Then

`\begin{gathered} A^(\prime)=(0*10,2*10)=(0,20) \\ A^(\prime)=(0,20) \end{gathered}`

Similarly, to get B'

`\begin{gathered} B^(\prime)=(3*10,-2*10) \\ B^(\prime)=(30,-20) \end{gathered}`

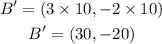
Finally to get C'

`\begin{gathered} C^(\prime)=(-1*10,-4*10) \\ C^(\prime)=(-10,-40) \end{gathered}`

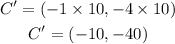
Finally