Step-by-step explanation
We are asked to solve the given quadratic equation
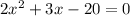
To do so, we will have to find the factors of the product of the coefficient of the first and last term whose sum is 3
Thus
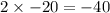
So the factor of -40 which also gives a sum of 3 will be

Thus

We will have the answer will be
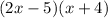
Simplifying further
we will have the zeros to be
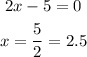
Also
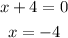
So the answers are:
x = -4 and x= 5 over 2