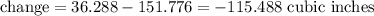If the length of the cut-out was "x" inches, then the length of the box is:

The width of the box is:
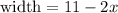
The height is:

The volume of the box is given by:
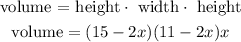
If the cutout was 0.6 inches. The volume is:

If the cutout was 2.4 inches.
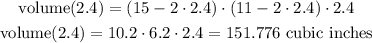
The change on the volume of the box is the difference between the two volumes above:
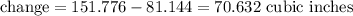
If the cutout was 4.8 inches.
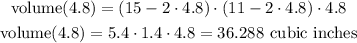
The change on the volume of the box is the difference between the volumes from when the cutout was 4.8 inches and 2.4 inches.