Velocity of that jet flying against the jetstream is:
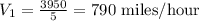
And the velocity of that jet flying with the jetstream is:
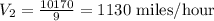
Let the velocity of that jet in still air be x miles per hour and the velocity of jetstream be y miles per hour, so:
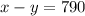
and
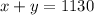
Adding the two equations we have:
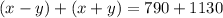
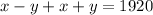

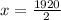
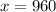
the velocity of that jet in still air is 960 miles per hour.
And the rate of the jetstream "y" is (replacing x in the first equation):
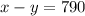
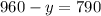
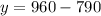
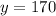
170 miles per hour