Concept
To find the values of x and y, you apply the trigonometry ratio formula.

Step 1: Name the given sides
Opposite = 5 side facing the given angle 45 degrees
Hypotenuse = x side facing the right angle
Adjacent = y the third side
Step 2: Substitute the values of the sides to find the unknown.
![\begin{gathered} \text{From} \\ \sin \theta\text{ = }\frac{Opposite}{\text{Hypotenuse}} \\ \sin 45\text{ = }(5)/(x) \\ \frac{1}{\sqrt[]{2}}\text{ = }(5)/(x) \\ \text{Cross multiply} \\ x\text{ = 5}\sqrt[]{2} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/te868uwukxlzjnjqphzvo230qu0us3jbpx.png)
Step 3: find the value of y using tangent angle.
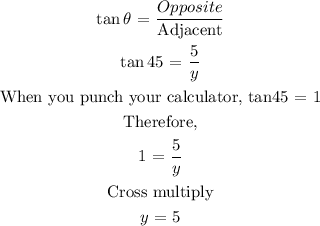
Final answer
Option B