Solution:
Given the system of inequalities:
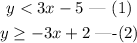
From inequality (1),
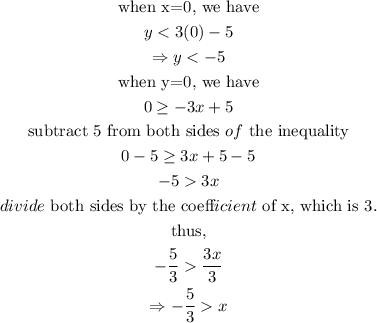
From inequality (2),
![\begin{gathered} \text{when x=0, we have} \\ y\ge-3(0)+2 \\ \Rightarrow y\ge2 \\ \text{when }y=0,\text{ we have} \\ 0\ge-3x+2 \\ \text{subtract }2\text{ from both sides of the inequality} \\ 0-2\ge-3x+2-2 \\ \Rightarrow-2\ge-3x \\ \text{divide both sides by the coefficient of x, which is -3.} \\ \text{thus,} \\ -(2)/(-3)\ge-(3x)/(-3) \\ \Rightarrow x\le(2)/(3) \\ \text{Note: when the coefficient of the unknown is negative, the inequality sign changes when dividing.} \end{gathered}]()
Thus, plotting these range of values of x and y on a graph, we have
In the above plot, the intersections of the shaded regions of the inequalities is the solution to the system of inequalities.