The given figure
There is a circle of radius of 10
Then the hypotenuse of the right triangle is the radius of the circle = 10
Since one leg of the triangle is 6
Then we can use the Pythagoras Theorem to find AE, then multiply it by 2 to find AB
Since < AEM = 90 degrees, then
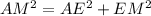
M is the center of the circle
Since AM = 10, ME = 6, then
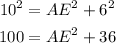
Subtract 36 from both sides
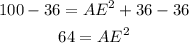
Take a square root for both sides
![\begin{gathered} \sqrt[]{64}=\sqrt[]{AE^2} \\ 8=AE \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/jas851egdetcf8eexxkrd2kjmy8uo638p7.png)
Since DC is perpendicular to AB and passing through the center of the circle, then
DC bisects AB, which means E is the mid-point of AB
AE = EB = 8
AB = 8 + 8 = 16
AB = 16