Question

A plan for a garden is drawn in the coordinate plane. The garden is in the shape of a trapezoid. Each unit in the coordinate plane represents 1 foot what is the total area of the garden?

Answer 1

It is given that,

The garden is in the shape of a trapezoid

From the given figure,

The length of the bottom of the trapezoid,

`=10-(-10)`
`=10+10=20`

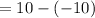
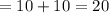
The length of the bottom of the trapezoid is 20 feet

The length of the top of the trapezoid is,

`=6-(-6)=12`

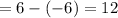
The length of the two parallel sides of the trapezoid are 20 feet and 12 feet.

To find the height of the trapezoid

Height of the trapezoid is,

`=2-(-4)=2+4=6`

Height of the trapezoid is 6

To find the area of the trapezoid

we know that,

Area of the trapezoid is

`=(1)/(2)*(a+b)*(h)`

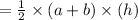
where a and b are the paralell sides of the trapezoid and h is the height of the trapezoid.

Substitute the values we get,

Area of the trapezoid is

`=(1)/(2)*(20+12)*6`
`=(1)/(2)*32*6=96`

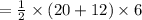
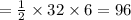
Area of the trapezoid is 96 feet.