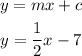Question

What equation would allow you to land this plane on the runway

Answer 1

To find the equation that represent the land of the plane (the blue line) we have to find the slope and the interseccion with the y axis.

So in the graph we can see that the intersection is:

`c=-7`

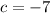
Now to find the slope que can use the green points that made a parallel line to the blue line, so with the coordinates (8,-2) and (10,-1) we can use this equation:

`m=(y_2-y_1)/(x_2-x_1)`

and we replace the coordinates so:

`m=(-1-(-2))/(10-8)=(1)/(2)`

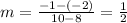
So the final equation will be:

`\begin{gathered} y=mx+c \\ y=(1)/(2)x-7 \end{gathered}`