Question

Solve completing the square-4x2 +32x -60 = 0divide by -4 first

Answer 1

Solving an equation by completing the square

Having the following equation:

-4x² + 32x - 60 = 0

we want to solve it

Step 1: we divide both sides by -4

`(-4x^2+32x-60)/(-4)=(0)/(4)=0`

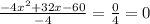
we distribute the division for each term

`\begin{gathered} (-4x^2)/(-4)+(32x)/(-4)+(-60)/(-4)=0 \\ x^2-8x+15=0 \end{gathered}`

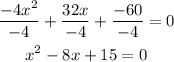
Step 2: completing the square

We want to transform the left side to the form:

`x^2-2ax+a^2`

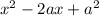
We are going to rearrange our equation so we have just the first and second term, in order to do so we substract 15 both sides:

`x^2-8x=-15`

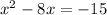
We want to express the second term, 8x, as a multiplication by 2, 2a. Since

2 · 4 = 8, then we have that a = 4.

Then

`\begin{gathered} x^2-8x \\ =x^2-2\cdot4x=15 \end{gathered}`

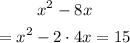
Since a² = 4² = 16, we are going to add both sides 16, so we have this equation with the form of the beggining of this step:

`\begin{gathered} x^2-2\cdot4x=15 \\ x^2-2\cdot4x+16=15+16 \\ x^2-2\cdot4x+4^2=31 \end{gathered}`

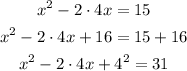
Step 3: solving the equation

We are going to factor the left side of the equation:

`\begin{gathered} x^2-2\cdot4x+4^2 \\ =\mleft(x-4\mright)^2=31 \end{gathered}`

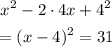
Now, we square root both sides:

`\begin{gathered} (x-4)^2=31 \\ x-4=\pm\sqrt[]{31} \end{gathered}`

Now, we add 4 both sides:

`x^{}=4\pm\sqrt[]{31}`

We have two answers now,

`\begin{gathered} x_1=4+√(31)\approx4+5.6=9.6 \\ x_2=4-\sqrt[]{31}\approx4-5.6=-1.6 \end{gathered}`