We have a principal of $2500 that has to be paid in 4 years. The payments will be monthly and all equals.
The annual interest rate is 18%.
Then, we can use the expression for an annuity that is paid monthly (m = 12, the number of superiods per year):
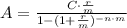
where A is the monthly payment, C is the principal ($2500), r is the interest rate (r = 0.18), m is the number of superiods per year (m = 12) and n is the number of periods related to the interest rate (n =4).
Then, we can replace and solve this as:

Answer: we have to pay $73.44 each month.