Question

An electron moving at 4.10 ✕ 103 m/s in a 1.45 T magnetic field experiences a magnetic force of 1.40 ✕ 10−16 N. What angle does the velocity of the electron make with the magnetic field? There are two answers between 0° and 180°. ° (smaller value) ° (larger value)

Answer 1

Step-by-step explanation

Parameters given:

Speed of electron, v = 4.10 * 10^3 m/s

Magnetic field, B = 1.45 T

Magnetic force, F = 1.40 * 10^(-16) N

To find the angle that the velocity of the electron makes with the magnetic field, apply the formula for magnetic force:

`F=qvB\sin\theta`

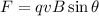
where θ = angle

q = electric charge = 1.6 * 10^(-19) C

Make θ the subject of the formula:

`\begin{gathered} \sin\theta=(F)/(qvB) \\ \\ \theta=\sin^(-1)((F)/(qvB)) \end{gathered}`

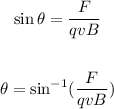
Therefore, the angle that the velocity makes is:

`\begin{gathered} \theta=\sin^(-1)((1.4*10^(-16))/(1.6*10^(-19)*4.1*10^3*1.45)) \\ \\ \theta=\sin^(-1)(0.1472) \\ \\ \theta=8.46\degree \end{gathered}`

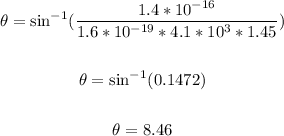
To find the second angle, subtract the angle from 180 degrees:

`\begin{gathered} 180-8.46 \\ \\ 171.54\degree \end{gathered}`

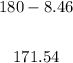
The angles are: