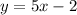Question

(1,3) and (0,-2) written in slope intercept form

Answer 1

We are given the following points:

`\begin{gathered} \mleft(1,3\mright) \\ \mleft(0,-2\mright) \end{gathered}`

To determine the slope-intercept form of the equation we need to use the following general form of a line equation:

`y=mx+b`

Where "m" is the slope and "b" is the y-intercept. The value of the slope is given by the following formula:

`m=(y_2-y_1)/(x_2-x_1)`

Where:

`\begin{gathered} (x_1,y_1)=(1,3) \\ (x_2,y_2)=(0,-2) \end{gathered}`

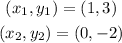
Replacing in the formula for the slope:

`m=(-2-3)/(0-1)`

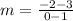
Solving the operations:

`m=-(5)/(-1)=5`

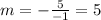
Replacing the value of the slope:

`y=5x+b`

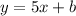
Now we replace the point (x,y) = (0,-2) to get the value of "b":

`\begin{gathered} -2=5(0)+b \\ -2=b \end{gathered}`

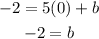
Replacing in the equation of the line:

`y=5x-2`