Answer:
The slope of the line between the two points is;
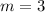
the slope-intercept form of the line joining the two points is;

Step-by-step explanation:
Given the points;

1.
We want to find the slope, using the coordinates;

The slope of the line between the two points is;
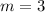
2.
Writing the equation in slope-intercept form;

Let us substitute the slope and the coordinates of a point into the point-slope form of a linear equation;

Therefore, the slope-intercept form of the line joining the two points is;
