Question

Is triangle ABC: A(2,-1), B(5,-1) C(5,3) a right triangle? Explain.

Answer 1

Given:

A(2, -1), B(5, -1), C(5, 3)

Let's determine the if the triangle ABC is a right triangle.

To determine if it is a right triangle, let's find the length of each side by using distance formula, then apply Pythagorean Theorem.

Apply the distance formula:

`d=\sqrt[]{(x2-x1)^2+(y2-y1)^2}`

Thus, we have:

Length of AB:

A(2, -1), B(5, -1)

`\begin{gathered} AB=\sqrt[]{(5-2)^2+(-1-(-1))^2} \\ \\ AB=\sqrt[]{(3)^2+(0)^2}=\sqrt[]{3^2}=3 \end{gathered}`

Length of BC:

B(5, -1), C(5, 3)

`\begin{gathered} BC=\sqrt[]{(5-5)^2+(3-(-1))^2} \\ \\ BC=\sqrt[]{(0)^2+(3+1)^2}=\sqrt[]{0+4^2}=4 \end{gathered}`

Length of AC:

A(2, -1), C(5, 3)

`\begin{gathered} AC=\sqrt[]{(5-2)^2+(3-(-1))^2} \\ \\ AC=\sqrt[]{(3)^2+(3+1)^2} \\ \\ AC=\sqrt[]{9+16}=√(25)=5 \end{gathered}`

Thus, we have the following side lengths of triangle ABC:

AB = 3

BC = 4

AC = 5

APply Pythagorean Theorem:

`c^2=a^2+b^2`

Where:

a = 3

b = 4

c = 5

Let's verify if the equation is true:

`\begin{gathered} c^2=a^2+b^2 \\ \\ 5^2=3^2+4^2 \\ \\ 25=9+16 \\ \\ 25=25 \end{gathered}`

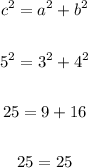
SInce the left hand side equals the right hand side, the equation is true.

Therefore, the triangle ABC is a right triangle.

ANSWER:

Yes, the triangle ABC is a right triangle.