Question

How would I solve this? Im having a lot of trouble. Thank you.Part A

Answer 1

2.29 rad

Explanation:

The terminal arm of an angle in standard position passes through the point:

`(-7,8)`

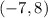
For an angle in standard position, its angle in degrees:

`\begin{gathered} \theta=\arctan\left((y)/(x)\right) \\ \implies\theta=\arctan\left((8)/(-7)\right)=-48.81 \end{gathered}`

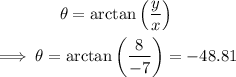
Since (-7, 8) is in Quadrant II:

`\theta=180-48.81=131.19\degree`

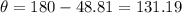
Finally, convert the result to radians:

`\theta=131.19*(\pi)/(180)=2.29\text{ rad}`

The radian value of the angle in the interval (0, 2π) correct to the nearest hundredth is 2.29 radians.