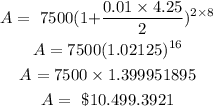Question

Please helppHow much money would you have after 7 years if you invested $12,000 at 2% compounded annually?

Answer 1

Step 1

write the expression for the amount compounded annually or in a given period of time and define the terms

`\begin{gathered} A=P(1+(r)/(100))^n \\ \text{where } \\ A=\text{ amount } \\ r\text{ = rate per year = 2\%} \\ n\text{ = time = 7 years} \\ P\text{ = principal = \$12,000} \end{gathered}`

Step 2

Substitute these values into the above equation and find the amount compounded annually after 7 years

`\begin{gathered} A\text{ = 12000(1+}(2)/(100))^7 \\ A\text{ = 12000(1+0.02})^7 \\ A=12000(1.02)^7 \\ A=\text{ 12000}*1.148685668 \\ A=\text{ \$13,784.22801} \end{gathered}`

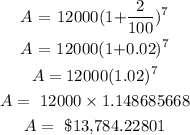
In 7 years, annually, he will compound $13,784.22801

For question 2

Use the formula

Substituting these values into the formula yields

`\begin{gathered} A=\text{ 7500(1+}(0.01*4.25)/(2))^(2*8) \\ A=7500(1.02125)^(16) \\ A=7500*1.399951895 \\ A=\text{ \$10.499.3921} \end{gathered}`