Question

A train whistle is heard at 330 Hz as the train approaches town. The train cuts its speed in half as it nears the station, and the sound of the whistle is then 320 Hz.Part AWhat is the speed of the train before slowing down?Part BWhat is the speed of the train after slowing down?

Answer 1

Let at velocity v the frequency is 330 Hz.

At velocity v/2 the frequency is 320 Hz.

Using Doppler effect,

`f_1=f_o(v_s)/(v_s-v)`
`f_2=f_o(v_s)/(v_s-(v)/(2))`

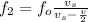
Dividing the equations,

`\begin{gathered} (f_1)/(f_2)=\frac{f_o((v_s)/(v_s-v))}{f_o(\frac{v_s}{v_s-(v)/(2)_{}_{}})_{}_{}} \\ \Rightarrow v=v_s(((f_1)/(f_2)-1))/(((f_1)/(f_2)-(1)/(2))) \end{gathered}`

Putting the values we have,

`\begin{gathered} v=(20.17m)/(s) \\ (v)/(2)=10.08\text{ m/s} \end{gathered}`

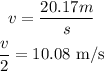
Thus, the speed of the train before slowing down is 20.17 m/s

The speed of the train after slowing down is 10.08 m/s