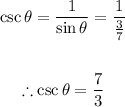Solution
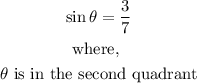
- If θ is in the second quadrant, then it means that θ is between 91° and 180°.
- Also, in the second quadrant, only sine and cosec are positive. tan (θ), cot (θ), sec(θ), cos (θ) are all negative in the second quadrant.
- Let us solve the question using this knowledge:
- Cos (θ)
![\begin{gathered} \sin \theta=\frac{Opposite}{\text{Hypotenuse}}=(3)/(7) \\ \\ \text{Opposite}^2+\text{Adjacent}^2=\text{Hypotenuse}^2 \\ 3^2+\text{Adjacent}^2=7^2 \\ 9+\text{Adjacent}^2=49 \\ \therefore\text{Adjacent}=\sqrt[]{40} \\ \\ \therefore\cos \theta=\frac{\sqrt[]{40}}{7}\text{ if }\theta\text{ is in the first quadrant} \\ \\ \cos \theta=-\frac{\sqrt[]{40}}{7}\text{ if }\theta\text{ is in the second quadrant} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/temtir7quvdqd52daq4m1p41e8nsheabw7.png)
- Tan (θ)
![\begin{gathered} \tan \theta=(\sin\theta)/(\cos\theta)=(3)/(7)/(-\frac{\sqrt[]{40}}{7}) \\ \\ \tan \theta=(3)/(7)*\frac{7}{-\sqrt[]{40}}=-\frac{3}{\sqrt[]{40}} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/6rhkg3v060ze75qnthbyxi2tch5uzvrubb.png)
Cot (θ)
![\begin{gathered} \cot \theta=(1)/(\tan \theta) \\ \\ \cot \theta=\frac{1}{-\frac{3}{\sqrt[]{40}}}=-\frac{\sqrt[]{40}}{3} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/jwr79w1x4ryze95v0xtoy24zbwh7sbvh57.png)
Sec (θ)
![\begin{gathered} \sec \theta=(1)/(\cos \theta) \\ \\ \sec \theta=\frac{1}{-\frac{\sqrt[]{40}}{7}}=-\frac{7}{\sqrt[]{40}} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/d48ali3rswet0tut9qxklqqu69bwznfyiu.png)
Csc (θ)