Question

A spring with a constant of 250 n/m is compressed .73 meters the spring is sitting parallel to a frictionless table top with a 1.5 KG block of wood pushed into it at point A what would be the maximum speed of the block at point B when does spring is allowed to spring

Answer 1

Given,

The spring constant of the spring, k=250 N/m

The compression of the spring, x=0.73 m

The mass of the block, m=1.5 kg

As the spring expands, it loses its potential energy. The potential energy lost by the spring is gained by the block in the form of kinetic energy, according to the law of conservation of energy.

Thus,

`(1)/(2)mv^2=(1)/(2)kx^2_{}`

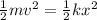
Where v is the maximum speed the block will have at point B.

On rearranging the above equation,

`\begin{gathered} v^2=(kx^2)/(m) \\ \Rightarrow v=\sqrt[]{(kx^2)/(m)} \end{gathered}`

On substituting the known values in the above equation,

`\begin{gathered} v=\sqrt[]{(250*0.73^2)/(1.5)} \\ =9.4\text{ m/s} \end{gathered}`

Thus the maximum speed of the block at point B will be 9.4 m/s

Therefore the correct answer is option 3.