Answer
Option A is correct.
y = 4x + 3
Step-by-step explanation
To do this, we need to know that
The slope and y-intercept form of the equation of a straight line is given as
y = mx + c
where
y = y-coordinate of a point on the line.
m = slope of the line.
x = x-coordinate of the point on the line whose y-coordinate is y.
c = y-intercept of the line.
So, we need to calculate the slope and y-intercept of this
For a straight line, the slope of the line can be obtained when the coordinates of two points on the line are known. If the coordinates are (x₁, y₁) and (x₂, y₂), the slope is given as

Taking the first two given values for (x₁, y₁) and (x₂, y₂)
(x₁, y₁) and (x₂, y₂) = (0, 3) and (2, 11)
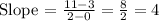
And the y-intercept is the value of y when x = 0
when x = 0, y = 3
So,
y = mx + c is
y = 4x + 3