Question

Find the range and standard deviation of the set of data.10, 10, 5, 12, 18 What is the range

Answer 1

Step 1

Find the range

Range = largest value - smallest value

Range = 18-5 =13

Step 2

`\begin{gathered} \text{Write an expression for the mean} \\ \bar{x}=(\Sigma x)/(n) \end{gathered}`

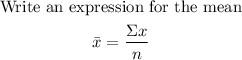
Where Σx = 10 +10+5+12+18 = 55

n = 5

Step 2

Substitute in and find the mean

`\bar{x}=(55)/(5)=11`

Hence the mean = 11

Step 4

Write an expression for the standard deviation

`\text{Standard deviation =}\sqrt[]{\fracx-\bar^2{n}}`

Step 5

Draw up a table to help find the standard deviation

Hence substituting yields

`\begin{gathered} sd=\sqrt[]{(88)/(5)} \\ sd=4.19524 \end{gathered}`

The standard deviation approximately = 4.20 to the nearest hundredth.