Question

If there are 360 grams of radioactive material with half-life (decrease by half or 50%)of 1 hour, how much of the radioactive material will be left after 4 hours?

Answer 1

We have here an exponential decay. In this case, we have to use the formula:

`y=a(1-r)^x^{}`

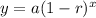
Where:

a = initial value (in this case, 360 grams)

r = decay rate (in this case, we have 50/100= 0.5.

x = number of time intervals that have passed (in this case, 4 hours).

Then, we have that:

`y=360\cdot(1-0.5)^4\Rightarrow y=300\cdot(0.5)^4\Rightarrow y=22.5`

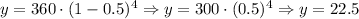
Hence, after four hours there will be 22.5 grams of the radioactive material.