Question

A grasshopper hops along a level road. On each hop, the grasshopper launches himself at an angle of 45⁰ and achieves a range of 0.80 m. What is the average horizontal speed of the grasshopper as it hops along the road?

Answer 1

First, let's decompose the grasshopper speed V in its horizontal and vertical velocities Vx and Vy:

`\begin{gathered} V_x=V\cdot\cos (45\degree) \\ V_x=V\cdot\frac{\sqrt[]{2}}{2} \\ \\ V_y=V\cdot\sin (45\degree) \\ V_y=V\cdot\frac{\sqrt[]{2}}{2} \\ \\ V_x=V_y=v \end{gathered}`

For the vertical movement, let's use the following formula for the change in speed during the whole movement. The initial vertical speed is v, and the final vertical speed is -v (same magnitude but opposite direction, when the grasshopper reaches the ground). The acceleration is the gravity acceleration (a = -9.8 m/s²).

`\begin{gathered} V=V_0+a\cdot t_{} \\ -v=v-9.8\cdot t \\ 2v=9.8t \\ v=4.9t \end{gathered}`

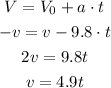
Now, for the horizontal movement, we have:

`\begin{gathered} \text{distance}=\text{speed}\cdot\text{time} \\ 0.8=v\cdot t \\ 0.8=4.9t\cdot t \\ 0.8=4.9t^2 \\ t^2=(0.8)/(4.9) \\ t^2=0.163265 \\ t=0.404\text{ s} \end{gathered}`

Calculating the average horizontal speed, we have:

`\begin{gathered} \text{speed}=\frac{\text{distance}}{\text{time}} \\ \text{speed}=(0.8)/(0.404) \\ \text{speed}=1.98\text{ m/s} \end{gathered}`

Therefore the average horizontal speed is 1.98 m/s.