Question

Hello, I need small help with math please and thank you

Answer 1

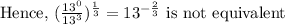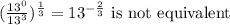
`((13^0)/(13^3))^{(1)/(3)}=13^{-(2)/(3)}\text{ is not equivalent}`Step-by-step explanation:
`\begin{gathered} \text{Given:} \\ ((13^0)/(13^3))^{(1)/(3)}=13^{-(2)/(3)} \\ (\frac{13^{}}{13^3})^{(1)/(3)}=(13^(-2))^{(1)/(3)} \end{gathered}`

To determine why the two expressions/statements are wrong, we need to solve each seperately and compare the result

`\begin{gathered} ((13^0)/(13^3))^{(1)/(3)}=13^{-(2)/(3)} \\ \text{left hand side of the equation: }((13^0)/(13^3))^{(1)/(3)} \\ \text{The base of the left side is common, we simplify by subtracting the exponents:} \\ (13^(0-3))^{(1)/(3)}=(13^(-3))^{(1)/(3)} \\ =13^{-(3)/(3)\text{ }}=13^(-1) \\ \\ \text{The result of the left hand side is not the }sa\text{me as the right hand side} \\ 13^(-1)\text{ }\\e\text{ }13^{-(2)/(3)} \end{gathered}`
`\begin{gathered} (\frac{13^{}}{13^3})^{(1)/(3)}=(13^(-2))^{(1)/(3)} \\ \text{left hand side: }(\frac{13^{}}{13^3})^{(1)/(3)} \\ (\frac{13^{}}{13^3})^{(1)/(3)}=(\frac{13^1^{}}{13^3})^{(1)/(3)} \\ \text{The base are common, subtract exponents as they are seperated by division:} \\ (13^(1-3))^{(1)/(3)}=(13^(-2))^{(1)/(3)} \\ =13^{-(2)/(3)} \\ \\ \text{right hand side:} \\ (13^(-2))^{(1)/(3)}=13^{-(2)/(3)} \\ \\ \text{The left hand side = right hand side} \\ \text{Hence, }(\frac{13^{}}{13^3})^{(1)/(3)}=(13^(-2))^{(1)/(3)} \end{gathered}`

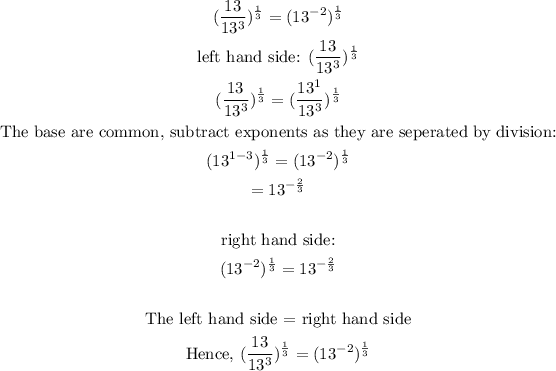
From our workings, we see the first equation statement is wrong so we cannot compare it with the other equation for equivalence

`\text{Hence, }((13^0)/(13^3))^{(1)/(3)}=13^{-(2)/(3)}\text{ is not equivalent}`