ANSWER
D. obtuse
Step-by-step explanation
We want to determine what type of triangle has the given sides.
To do this, we have to follow the rules given below:
1. If the square of the longest side is equal to the sum of the square of the other two sides, it is a right triangle.
2. If the square of the longest side is greater than the sum of the square of the other two sides, it is an obtuse triangle.
3. If the square of the longest side is less than the sum of the square of the other two sides, it is an acute triangle.
The longest side is 13. Its square is:
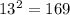
The other two sides are 12 and 2√3. The sum of their squares is:
![12^2+(2\sqrt[]{3})^2=156](https://img.qammunity.org/2023/formulas/mathematics/college/mzbkyxlo9fky1fwnjdyw3evrdh42m44foc.png)
Since the square of the longest side is greater than the sum of the square of the other two sides, it is an obtuse triangle.