Recall the rule that gives that the external angle of a triangle is equal to the sum of the two opposite interior angles. Consider the triangle below:
The rule applies and gives us:
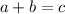
Applying the rule to the question, we have:
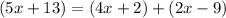
Solving, we can get x to be:

The value of x is 20.