2.69 miles
1) Gathering the data, let's sketch that:
2) As we can see we have two triangles, so we can solve it using trigonometry.
So we can write that the height of the mountain is equal to the following trigonometric ratios (tangent):
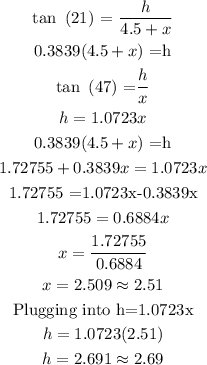
So the height of the mountain is approximately 2.69 miles