the investment after 3 years will be worth $73.21
Step-by-step explanation:
Amount invested = Principal
P = $55
n = number of time scompounded = annually
n = 1
rate = r = 10% = 0.1
FV = future value after 3 years
Using compound interest formula:
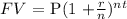
substituting the values in the formula:

Hence, the investment after 3 years will be worth $73.21