6. Given the expression
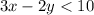
To plot this expression, first step is to write it in terms of y, that is, isolate the y term alone in one side of the expression and all other terms in the other side:

Next you have to follow the same procedure as when you are ploting a line, choose two values of x and replace them in the expression to calculate the corresponding values of y. Next plot the line that crosses all points.
For example
x=0

x= -5
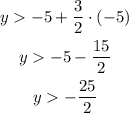
x=5

The three points are (5, 5/2), (0,-5) and (-5,-25/2)
Plot them and link them with a line. Once you have the line ploted, you have to shadow the area that represents the inequation.
Remember that the expression indicates the limit or start of the inequation.
For this particular expression, you have to shadow the area to the left of the line.