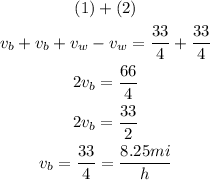Question

a boat travels 33 miles Downstream in 4 hours. The return trip takes the boat 4 hours. Find the speed of the boat in still water.

Answer 1

Let's use the following formula:

`v=(d)/(t)`

We have two speeds in this case, the speed of the boat and the speed of the water, so:

`\begin{gathered} v_b=Speed_{\text{ }}of_{\text{ }}the_{\text{ }}bo_{}at \\ v_w=Speed_{\text{ }}of_{\text{ }}the_{\text{ }}water \end{gathered}`

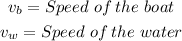
When the boat travels 33 miles downstream in 4 hours, we can say that their speeds add up:

`v_b+v_w=(33)/(4)`

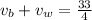
When the boat return, we can say that their speeds are subtracted:

`v_b-v_w=(33)/(4)`

with this we can form a system of equations 2x2:

`\begin{gathered} v_b+v_w=(33)/(4)_{\text{ }}(1)_{} \\ v_b-v_w=(33)/(4)_{\text{ }}(2) \end{gathered}`

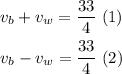
Let's solve for vb:

`\begin{gathered} (1)+(2) \\ v_b+v_b+v_w-v_w=(33)/(4)+(33)/(4) \\ 2v_b=(66)/(4) \\ 2v_b=(33)/(2) \\ v_b=(33)/(4)=(8.25mi)/(h) \end{gathered}`