Question

Need to know the area and shape type and drawing how its done

Answer 1

In order to determine the area of the shape, we see that a part of the shape is a rectangle and other part is a tiangle.

We know that for a retangle the area is defined by the product of it's sides and for a triangle it is defined by the height times it's base.

From this, we proceed to calculate the area for the rectangle:

`A_r=s_1\cdot s_2`

Here s1 is one of the sides of the triangle, and s2 is the other side of the triangle that is different of the previous one.

`A_r=5\cdot8\Rightarrow A=40`

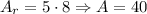
From this, we have the rectangle has an area of 40 ft^2.

Now, we proceed to calculate the area for the triangle:

`A_t=(b\cdot h)/(2)`

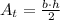
Here b is the base of the triangle and h is the height of the the triangle.

`A_t=(5\cdot2)/(2)\Rightarrow A_t=5`

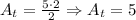
From this, we have that the area of the triangle is of 5 ft^2.

The total area of the shape is given by the sum of Ar and At, that is:

`A=A_r+A_t\Rightarrow A=40+5\Rightarrow A=45`

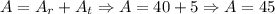
From this, we know that the total area of the shape is 45 ft^2.

Now, the graph:

Here the blue vertical line is the other end of the rectangle and is also the base of the triangle. The green horizontal line is the height of the triangle.