You have a triangle ABC with the following parameters:
a = ?
b = 12
c = 15
m∠A = 60
In order to calculate the value of side a, you use the Law of Cosines, which is given by the following formula:
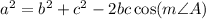
Then, by replacing into the previous equation you have:
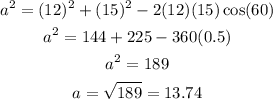
Hence, the value of the length of side a is 13.74