Answer:
√29/5
Explanation:
The terminal ray of ∠A passes through the point (10,−4).
From the diagram above, angle A is in quadrant IV.
First, find the value of r using the Pythagoras theorem:
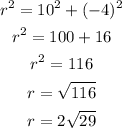
Secant is the inverse of cosine.
Therefore:

The value of sec A is √29/5.
Method 2
The terminal ray of ∠A passes through the point (10,−4).
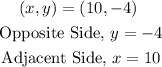
Find the value of the hypotenuse, r using the Pythagoras theorem:
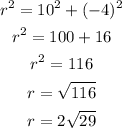
Secant is the inverse of cosine.
Therefore:

The value of sec A is √29/5.